Euler's identity
Appearance

Euler's identity, or Euler's equation, named after Leonhard Euler, is the equation of mathematical analysis
Quotes
[edit]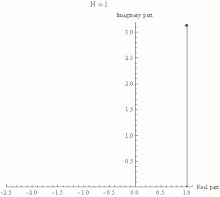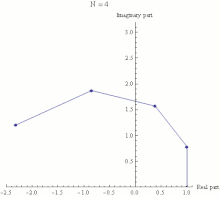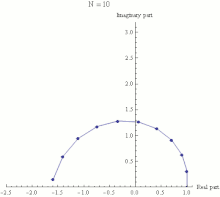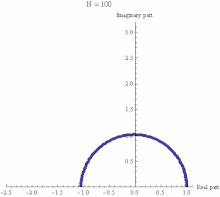
- One of the most frequently mentioned equations was Euler's equation, Respondents called it "the most profound mathematical statement ever written"; "uncanny and sublime"; "filled with cosmic beauty"; and "mind-blowing". Another asked: "What could be more mystical than an imaginary number interacting with real numbers to produce nothing?" The equation contains nine basic concepts of mathematics — once and only once — in a single expression. These are: e (the base of natural logarithms); the exponent operation; π; plus (or minus, depending on how you write it); multiplication; imaginary numbers; equals; one; and zero.
- Like a Shakespearean sonnet that captures the very essence of love, or a painting that brings out the beauty of the human form that is far more than just skin deep, Euler's equation reaches down into the very depths of existence.
- Keith Devlin, “The Most Beautiful Equation,” Wabash Magazine, Winter/Spring 2002. As quoted in Dr. Euler's Fabulous Formula : Cures Many Mathematical Ills (2006) ISBN 978-0691118222
- There is a famous formula, perhaps the most compact and famous of all formulas — developed by Euler from a discovery of de Moivre: It appeals equally to the mystic, the scientist, the philosopher, the mathematician.
- Edward Kasner and James R. Newman, in Mathematics and the Imagination (1940)

- Gentlemen, that is surely true, it is absolutely paradoxical; we cannot understand it, and we don't know what it means. But we have proved it, and therefore we know it must be the truth.
- Benjamin Peirce, as quoted in notes by W. E. Byerly, published in Benjamin Peirce, 1809-1880 : Biographical Sketch and Bibliography (1925) by R. C. Archibald; also in Mathematics and the Imagination (1940) by Edward Kasner and James Newman
- For any real number x, Euler’s formula is
- Where e is fundamental constant (the base of natural logarithms) and i = √-1. If we now put x =π, we get e^(iπ) = cos π+i sin π, and since cos(π) =−1 and sin(π) = 0, this reduces to e^(iπ) =−1 so that e^(iπ) +1=0.
- David Darling, in The Universal Book of Mathematics: From Abracadabra to Zeno's Paradoxes (2004), John Wiley & Sons, p. 111
- This most extraordinary equation first emerged in Leonard Euler’s Introductio in 1748.
- David Darling, in The Universal Book of Mathematics : From Abracadabra to Zeno's Paradoxes (2004), p. 111
- Since high school I have been utterly fascinated by the “mystery” of and by the beautiful calculations that flow, seemingly without end, from complex numbers and functions of complex variables.
- Paul J. Nahin, in Dr. Euler's Fabulous Formula: Cures Many Mathematical Ills (2011), Princeton University Press, p. xiii
- -1 = e^iπ,
proves that Euler was a sly guy.
- But ζ(2)
was totally new
- But ζ(2)
- And raised respect for him sky-high.
- William C. Waterhouse, as quoted in Dr. Euler's Fabulous Formula : Cures Many Ills (2011), by Paul J. Nahin, p. xiii
External links
[edit]





